M. Schilling catalog
Cheese-shaped surface of constant positive Gaussian curvature
Geodesic lines–Surfaces of revolution of constant Gaussian curvature
In the given above parametrization, the tangents to the profile curve at the end-points $t=\pm\pi/2$ are horizontal. Thus after rotation the resulting two holes in the surface can be glued up smoothly ($C^{1,1}$-smoothly, in fact) with two discs. The resulting surface looks like a head of cheese (illustration from W. Blaschke, Kreis und Kugel, de Gruyter, 1956):
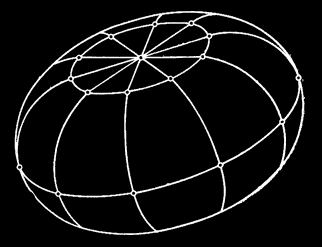
The model shows several heads of a cheese-shaped surface stack on each other.













